All Lessons
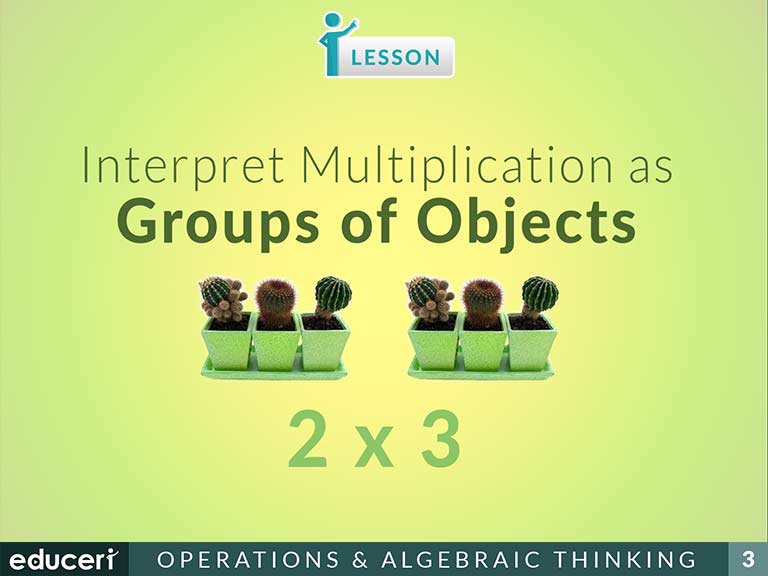
Interpret Multiplication as Groups of Objects
This operations and algebraic thinking lesson covers how to interpret multiplication as repeated addition of groups of equal amounts. The lesson includes research-based strategies and strategic questions that prepare students for assessments. In this lesson, students will examine word and picture problems to determine the number of groups and amount in each group. Then, they will select or create which situation can be solved using given multiplication statements.
Share This Lesson

Memorize Multiplication Facts
This operations and algebraic thinking lesson covers how to memorize multiplication facts. The lesson includes research-based strategies and strategic questions that prepare students for assessments. In this lesson, students will practice the multiplication facts of numbers 2 through 10 by a combination of reading aloud and writing each fact many times in horizontal and vertical formats.
Share This Lesson

Use Equal Sharing
This number sense lesson focuses on using equal sharing. The lesson includes research-based strategies and questions that help prepare students for assessments. In this lesson, students identify the first letter of each person's name in the problem, and use the letters to make a tally table. Then, they share the objects by giving one to each person equally, crossing out the object and adding a tally mark for that person. Finally, students write their answer in a complete sentence. In addition to the lesson, there are four pages of Independent Practice and review with questions modeled after current adaptive testing items.
Share This Lesson

Memorize the Multiplication Facts for 2
This number sense lesson focuses on memorizing multiplication facts for 2. The lesson includes research-based strategies and questions that help prepare students for assessments. In this lesson, students practice reading aloud, writing/filling in the product, and writing the entire multiplication fact for the number 2. In addition to the lesson, there are four pages of Independent Practice and review with questions modeled after current adaptive testing items.
Share This Lesson

Memorize the Multiplication Facts for 5
This number sense lesson focuses on memorizing multiplication facts for 5. The lesson includes research-based strategies and questions that help prepare students for assessments. In this lesson, students practice reading aloud, writing/filling in the product, and writing the entire multiplication fact for the number 5. In addition to the lesson, there are four pages of Independent Practice and review with questions modeled after current adaptive testing items.
Share This Lesson

Memorize the Multiplication Facts for 10
This number sense lesson focuses on memorizing multiplication facts for 10. The lesson includes research-based strategies and questions that help prepare students for assessments. In this lesson, students practice reading aloud, writing/filling in the product, and writing the entire multiplication fact for the number 10. In addition to the lesson, there are four pages of Independent Practice and review with questions modeled after current adaptive testing items.
Share This Lesson

Use the Associative Property of Multiplication
This algebra and functions lesson focuses on using the associative property of multiplication. The lesson includes research-based strategies and questions that help prepare students for assessments. In this lesson, students multiply the first two factors in column A, then multiply that product by the remaining factor. Then, they repeat they repeat the steps for column B. Finally, students compare the final products in column A and B. In addition to the lesson, there are four pages of Independent Practice and review with questions modeled after current adaptive testing items.
Share This Lesson

Memorize Division Facts 1-10
(J) Determine a quotient using the relationship between multiplication and division
4.4.F(F) Use strategies and algorithms, including the standard algorithm, to divide up to a four-digit dividend by a one-digit divisor
3.OA.7 Fluently multiply and divide within 100, using strategies such as the relationship between multiplication and division (e.g., knowing that 8 × 5 = 40, one knows 40 ÷ 5 = 8) or properties of operations. By the end of Grade 3, know from memory all products of two one-digit numbers.
4.NBT.64.NBT.6 Find whole-number quotients and remainders with up to four-digit dividends and one-digit divisors, using strategies based on place value, the properties of operations, and/or the relationship between multiplication and division. Illustrate and explain the calculation by using equations, rectangular arrays, and/or area models.
Share This Lesson

Memorize Multiplication Facts (Products over 45)
This Common Core operations and algebraic thinking lesson covers how to memorize multiplication facts. The lesson includes research-based strategies and strategic questions that prepare students for Common Core assessments. In this lesson, students will practice the multiplication facts of numbers with products over 45 by a combination of reading aloud and writing each fact many times in horizontal and vertical formats. In addition to the lesson, Independent Practice provides practice with random problems for each number, while periodic review provides practice with random problems for all the numbers together. The intent of this lesson is to provide additional practice for the multiplication facts that are more difficult for students to remember.
Share This Lesson

Solve Two-Step Word Problems (Addition & Subtraction)
Solve two-step word problems using addition and subtraction. Represent these problems using equations with a letter standing for the unknown quantity. Assess the reasonableness of answers using mental computation and estimation strategies including rounding.
Share This Lesson

Interpret Division as Groups of Objects
Interpret whole-number quotients of whole numbers, e.g., interpret 56 ÷ 8 as the number of objects in each share when 56 objects are partitioned equally into 8 shares, or as a number of shares when 56 objects are partitioned into equal shares of 8 objects each. For example, describe a context in which a number of shares or a number of groups can be expressed as 56 ÷ 8.
Share This Lesson
Use Multiplication Patterns
Apply properties of operations as strategies to multiply and divide. (Students need not use formal terms for these properties.) Examples: If 6 × 4 = 24 is known, then 4 × 6 = 24 is also known. (Commutative property of multiplication.) 3 × 5 × 2 can be found by 3 × 5 = 15, then 15 × 2 = 30, or by 5 × 2 = 10, then 3 × 10 = 30. (Associative property of multiplication.)







